Ncert Exercises & Solutions

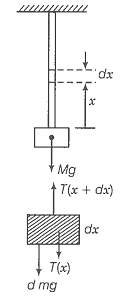
Q.25 (1) A steel wire of mass $μ$ per unit length with a circular cross-section has a radius of 0.1 cm. The wire is of length 10 m when measured lying horizontal, and hangs from a hook on the wall. A mass of 25 kg is hung from the free end of the wire. Assuming, the wire to be uniform and lateral strains << longitudinal strains, find the extension in the length of the wire. The density of steel is 7860 kg m^-3. (Young's modulus, Y = 2 x 10¹¹Nm^-2.

(2) If the yield strength of steel is 2.5 x 10⁸Nm^-2, what is the maximum weight that can be hung at the lower end of the wire?

Hint: Use the concept of Young's modulus to find the change in the length of the wire.

Consider the diagram. A small element of length dx is considered at x from the load (x=0).

Step 1: Find the tension in the wire.

(a) Let T(x) and T(x+dx) are tensions on the two cross-sections at distance dx apart, then

T (x+dx) - T(x) = dmg = $μ$ dxg (where

μ

is the mass/length). (

∵

dm =

μ

dx)

dT = $μ$ dxg [

∵

dT = T(x+dx) - T(x)]

$\Rightarrow$ T(x) = $μ$ gx + C (on integrating)

At x = 0, T(0) = Mg $\Rightarrow$ C = Mg

Step 2: Find the change in length of the wire.

Let the length dx at x increases by dr, then,

Young's modulus

Y = \frac{Stress}{Strain}

\frac{T (x) / A}{d r / d x} = Y

\Rightarrow \frac{d r}{d x} = \frac{1}{Y A} T (x)

\Rightarrow r = \frac{1}{Y A} \int_{0}^{L} (μ g x + M g) d x = \frac{1}{Y A} {[\frac{μ g x^{2}}{2} + M g x]}_{0}^{L} = \frac{1}{Y A} [\frac{m g L}{2} + M g L]

(m is the mass of the wire)

A = π \times {(10^{- 3})}^{2} m^{2}

Y = 200 \times 10^{9} {Nm}^{- 2}

m = π \times {(10^{- 3})}^{2} \times 10 \times 7860 kg

r = \frac{1}{2 \times 10^{11} \times π \times 10^{- 6}} [\frac{π \times 786 \times 10^{- 3} \times 10 \times 10}{2} + 25 \times 10 \times 10]

= [196.5 \times 10^{- 6} + 3.98 \times 10^{- 3}] = 4 \times 10^{- 3} m

Step 3: Find the maximum tension in the wire.

(b) Clearly tension will be maximum at x=L

∴ T = μ g L + M g = (m + M) g

[

∵

m =

μ

The yield force = (Yield strength Y x area = 250 x 10⁶ x

π

x (10^-3)²= 250 x

π

N
At yield point, T = Yield force

\Rightarrow

(m + M) g = 250 \times π

m = π \times {(10^{- 3})}^{2} \times 10 \times 7860 < < M

∴ M g = 250 \times π

Hence,

M = \frac{250 \times π}{10} = 25 \times π = 75 kg .

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions