(a) Let a drop of a liquid L be poured on a solid surface S placed in air A. If T_SL, and T_SA be the surface tensions corresponding to solid-liquid layer, liquid-air layer, and solid-air layer respectively and θ be the angle of contact between the liquid and solid, then

$T_{LA} \cos \theta +T_{SL}=T_{SA}$
$\Rightarrow \cos \theta =\frac{T_{SA}-T_{SL}}{T_{LA}}$
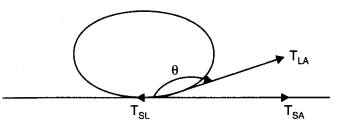
For the mercury-glass interface, T_SA< T_SL. Therefore, $\cos \theta$ is negative. Thus θ is an obtuse angle. For the water-glass interface, T_SA > T_SL. Therefore, $\cos \theta$ is positive. Thus, θ is an acute angle.

(b) Water wets glass because the force of cohesion of water is much less than the force of adhesion due to glass. In the case of mercury force of cohesion due to mercury molecules is quite strong as compared to the adhesion force due to glass. So, mercury does not wet glass and tends to create drops.

(c) Surface tension of liquid can be defined as the force acting per unit length on a line drawn tangentially to the liquid surface at rest. Since force is independent of the area of the liquid surface therefore, surface tension is also independent of the area of the liquid surface.

(d) As we know the clothes have narrow pores or spaces which act as capillaries. And the rise of liquid in a capillary tube is directly proportional to cosθ (Here θ is the angle of contact). As θ is small for detergent, therefore cos θ will be large. Due to this, the detergent will penetrate more in the narrow pores of the clothes.

(e) Since, any system tries to remain in a state of minimum energy. In the absence of any external force for a given volume of liquid, its surface area and surface energy is least for a spherical shape. It is due to this reason that a liquid drop, in the absence of an external force is spherical in shape.