Ncert Exercises & Solutions

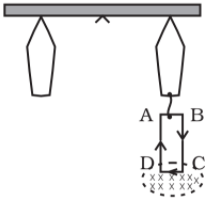
A 100 turn rectangular coil ABCD (in XY plane) is hung from one arm of a balance (Figure). A mass 500g is added to the other arm to balance the weight of the coil. A current 4.9 A passes through the coil and a constant magnetic field of 0.2 T acting inward (in x - z plane) is switched on such that only arm CD of length 1 cm lies in the field. How much additional mass ‘m’ must be added to regain the balance?

Hint: The total mass balances the magnetic force and the weight of the coil.

Step 1: For equilibrium/ balance, net torque should also be equal to zero.
When the field is off

\sum τ = 0

considering the separation of each hung from midpoint be l.

\begin{array}{l} Mgl = W_{coil} l \\ 0.5 gl = W_{coil} l \\ W_{coil} = 0.5 \times 9 .8 N \end{array}

Taking a moment of a force about mid-point, we have the weight of coil
Step 2: When the magnetic field is switched on:

\begin{array}{l} Mgl + mgl = W_{coil} l + IBLsin 90^{\circ} \times l \\ mgl = BIL \times l \\ m = \frac{BIL}{g} = \frac{0 .2 \times 4 .9 \times 1 \times 10^{- 2}}{9 .8} = 10^{- 3} kg = 1 g \end{array}

Thus, 1g of additional mass must be added to regain the balance.

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions