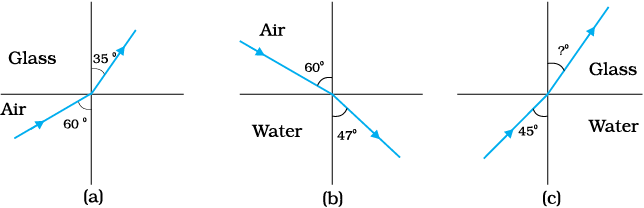
Hint: Apply snell's law

Step 1: Find the refractive index of glass.
For the glass-air interface:
Angle of incidence, i =60°
Angle of refraction, r=35°
The relative refractive index of the glass with respect to air,
${\mu }_{ga}=\frac{\sin i}{\sin r}=\frac{\sin {60}^{\circ }}{\sin {35}^{\circ }}$      

Step 2: Find the refractive index of water.
For the air-water interface:
The angle of incidence, i=$60°$
The angle of refraction, r=$47°$
$The\; relative\; refractive\; index\; of\; the\; water\; with\; respect\; to\; air,$
$\mu wa=sinisinr=sin60\circ sin47\circ$

Step 3: Find the refractive index of glass with respect to water.
The relative refractive index of glass with respect to water:
\(\mu_{gw}=\frac{\mu_{ga}}{\mu_{wa}}=\frac{\frac{sin60^{0}}{sin35^{0}}}{\frac{sin60^{0}}{sin47^{0}}}=\frac{sin47^{0}}{sin35^{0}}\)

Step 4: Find the angle of refraction at the water-glass interface.
For the glass-water interface:
The angle of incidence, i= $45°$
Let the angle of refraction = r
From Snell’s law,
${\mu }_{gw}=\frac{\sin i}{\sin r}$
$\Rightarrow \frac{\sin 47°}{\sin 35°}=\frac{\sin {45}^{\circ }}{\sin r}$
$\Rightarrow \sin r=\frac{{\displaystyle \frac{1}{\sqrt{2}}}}{1.275}=0.5546$
$\Rightarrow r={\sin }^{-1}\left(0.5546\right)=38.{68}^{\circ }$
Hence, the angle of refraction at the water-glass interface is 38.68°.