Hint: Use Snell's law.

Step 1: Find the optical path difference.

In this figure, we have shown a dielectric film of thickness d deposited on a glass lens.

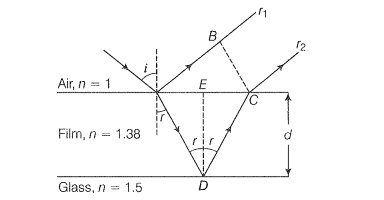
Refractive index of film = 1.38 and refractive index of glass = 1.5
Given, $\lambda =5500 \stackrel{\circ }{A}$

Consider a ray incident at an angle i. A part of this ray is reflected from the air-film interface and a part is refracted inside. This is partly reflected at the film-glass interface and a part transmitted. A part of the reflected ray is reflected at the film-air interface and a part transmitted as $r_2$ parallel to $r_1$. Of course, successive reflections and transmissions will keep on decreasing the amplitude of the wave.

Hence, rays $r_1$and r${}_2$ shall dominate the behaviour. If the incident light is to be transmitted through the lens, $r_1$ and $r_2$ should interfere destructively. Both the reflections at A and D are from lower to higher refractive index and hence, there is no phase change on reflection. The optical path difference between $r_2$ and $r_1$ is n(AD+CD)-AB.

lf d is the thickness of the film, then;

                                         $\begin{array}{l}AD=CD=\frac{d}{\cos r}\\ AB=AC\sin i\\ \frac{AC}{2}=d\tan r\\ AC=2d\tan r\end{array}$

Hence, AB=$2d\tan r\sin i$.

 $\begin{array}{l}Thus, the optical path difference==\frac{2nd}{\cos r}-2d\tan r\sin i\\ =2\cdot \frac{\sin i\times d}{\sin r\times \cos r}-2d\frac{\sin r}{\cos r}\sin i\\ =2d\sin i\left[\frac{1-{\sin }^{2}r}{\sin r\cos r}\right]\\ =2nd\cos r\end{array}$

Step 2: Find the conditions for maxima and minima.

For these waves to interfere destructively path difference $=\frac{\lambda }{2}$

$$
$\Rightarrow 2nd \cos r=\frac{\lambda }{2}$
$\Rightarrow nd \cos r =\frac{\lambda }{4}$
$$

For photographic lenses, the sources are normally in the vertical plane,

$\begin{array}{rl}\therefore i& =r=0^{\circ }\\ \text{From Eq. (i), nd}\cos 0^{\circ }& =\frac{\lambda }{4}\\ \Rightarrow d& =\frac{\lambda }{4n}\\ d& =\frac{5500\text{Å}}{4\times 1.38}\approx 1000\text{Å}\end{array}$