Ncert Exercises & Solutions

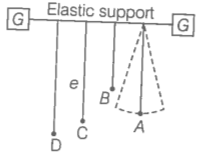
Four pendulums \(A,B,C,\) and \(D\) are suspended from the same elastic support as shown in the figure. \(A\) and \(C\) are of the same length, while \(B\) is smaller than \(A\) and \(D\) is large than \(A.\) If \(A\) is given a transverse displacement,

1.	\(D\) will vibrate with maximum amplitude.
2.	\(C\) will vibrate with maximum amplitude.
3.	\(B\) will vibrate with maximum amplitude.
4.	All four will oscillate with equal amplitude.

2. Hint: The amplitude of the pendulums depends on their frequencies.

Step 1: Find the comparative frequency of the pendulum.

According to the question, A is given a transverse displacement.

Through the elastic support, the disturbance is transferred to all the pendulums.

A and C are having the same length, hence they will be in resonance because of their time period of oscillation.

T = 2 π \sqrt{\frac{l}{g}}

and hence, the frequency is the same.

So, the amplitude of A and C will be maximum.

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions