Ncert Exercises & Solutions

4.30 A fighter plane flying horizontally at an altitude of 1.5 km with a speed 720 km/h passes directly overhead an anti-aircraft gun. At what angle from the vertical should the gun be fired for the shell with muzzle speed 600 m s-1 to hit the plane? At what minimum altitude should the pilot fly the plane to avoid being hit? (Take g = 10 m s-2 ).

NEETprep Answer:

$H e i g h t o f t h e p l a n e = 1.5 k m = 1500 m$
$S p e e d o f t h e p l a n e = 720 k m / h = 200 m / s$
$L e t θ b e t h e a n g l e w i t h t h e v e r t i c a l a s s h o w n i n t h e f i g u r e .$

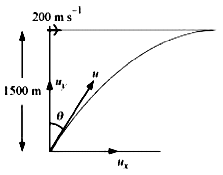
Muzzle velocity of the gun, u = 600 m/s

Time taken by the shell to hit the plane = t

Horizontal distance travelled by the shell = ut

Distance travelled by the plane = vt

The shell hits the plane. Hence, these two distances must be equal. $\begin{array}{l} u \sin θ = v \\ \sin θ = \frac{v}{u} \\ = \frac{200}{600} = \frac{1}{3} = θ .33 \\ \begin{matrix} θ = \sin^{- 1} (0.33) \\ = {19.5}^{\circ} \end{matrix} \end{array}$

In order to avoid being hit by the shell, the pilot must fly the plane at an altitude (H) higher than the maximum height achieved by the shell.

$H = \frac{u^{2} \sin^{2} (90 - θ)}{2 g}$
$= \frac{{(600)}^{2} \cos^{2} θ}{2 g}$
$= \frac{360000 x \cos^{2} 19.5}{2 x 10}$
$= 18000 x {(0.943)}^{2}$
$= 16006.482 m$
$= 16 km$

NEET Information

courses

Company

Botany Questions

Chemistry Questions

Physics Questions

Zoology Questions