We know that a capacitor and an inductor can store electrical and magnetic energy, respectively. When a capacitor (initially charged) is connected to an inductor, the charge on the capacitor and the current in the circuit exhibit the phenomenon of electrical oscillations similar to oscillations in mechanical systems (Chapter 14, Class XI).
Let a capacitor be charged qm (at t = 0) and connected to an inductor as shown in Fig. 7.18.
The moment the circuit is completed, the charge on the capacitor starts decreasing, giving rise to current in the circuit. Let q and i be the charge and current in the circuit at time t. Since di/dt is positive, the induced emf in L will have polarity as shown, i.e., vb < va. According to Kirchhoff’s loop rule,
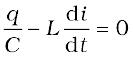 (7.39)
(7.39)
i = – (dq/dt) in the present case (as q decreases, i increases). Therefore, Eq. (7.39) becomes:
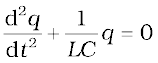 (7.40)
(7.40)
This equation has the form 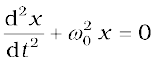 for a simple harmonic oscillator. The charge on the capacitor, therefore, oscillates with a natural frequency
for a simple harmonic oscillator. The charge on the capacitor, therefore, oscillates with a natural frequency
 (7.41)
(7.41)
and varies sinusoidally with time as
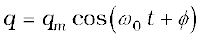 (7.42)
(7.42)
where qm is the maximum value of q and φ is a phase constant. Since q = qm at t = 0, we have cos φ =1 or φ = 0. Therefore, in the present case,
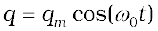 (7.43)
(7.43)
The current 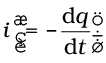 is given by
is given by
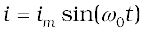 (7.44)
(7.44)
where 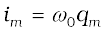
Let us now try to visualise how this oscillation takes place in the circuit.

Figure 7.19(a) shows a capacitor with initial charge qm connected to an ideal inductor. The electrical energy stored in the charged capacitor is 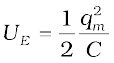 . Since, there is no current in the circuit, energy in the inductor is zero. Thus, the total energy of LC circuit is,
. Since, there is no current in the circuit, energy in the inductor is zero. Thus, the total energy of LC circuit is,
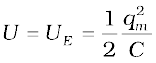
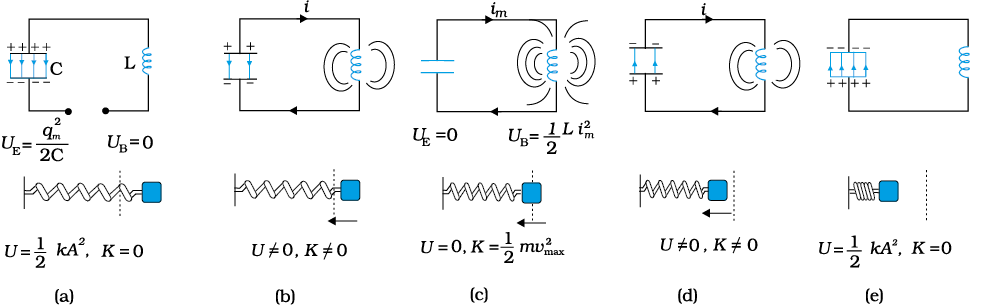
Figure 7.19 The oscillations in an LC circuit are analogous to the oscillation of a block at the end of a spring. The figure depicts one-half of a cycle.
At t = 0, the switch is closed and the capacitor starts to discharge [Fig. 7.19(b)]. As the current increases, it sets up a magnetic field in the inductor and thereby, some energy gets stored in the inductor in the form of magnetic energy: UB = (1/2) Li2. As the current reaches its maximum value im, (at t = T/4) as in Fig. 7.19(c), all the energy is stored in the magnetic field: . You can easily check that the maximum electrical energy equals the maximum magnetic energy. The capacitor now has no charge and hence no energy. The current now starts charging the capacitor, as in Fig. 7.19(d). This process continues till the capacitor is fully charged (at t = T/2) [Fig. 7.19(e)]. But it is charged with a polarity opposite to its initial state in Fig. 7.19(a). The whole process just described will now repeat itself till the system reverts to its original state. Thus, the energy in the system oscillates between the capacitor and the inductor.
The LC oscillation is similar to the mechanical oscillation of a block attached to a spring. The lower part of each figure in Fig. 7.19 depicts the corresponding stage of a mechanical system (a block attached to a spring). As noted earlier, for a block of a mass m oscillating with frequency ω0, the equation is
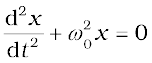
Here, 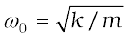 , and k is the spring constant. So, x corresponds to q. In case of a mechanical system F = ma = m (dv/dt) = m (d2x/dt2). For an electrical system, ε = –L (di/dt) = –L (d2q/dt2). Comparing these two equations, we see that L is analogous to mass m: L is a measure of resistance to change in current. In case of LC circuit,
, and k is the spring constant. So, x corresponds to q. In case of a mechanical system F = ma = m (dv/dt) = m (d2x/dt2). For an electrical system, ε = –L (di/dt) = –L (d2q/dt2). Comparing these two equations, we see that L is analogous to mass m: L is a measure of resistance to change in current. In case of LC circuit, 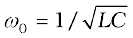 and for mass on a spring,
and for mass on a spring, 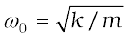 . So, 1/C is analogous to k. The constant k (=F/x) tells us the (external) force required to produce a unit displacement whereas 1/C (=V/q) tells us the potential difference required to store a unit charge. Table 7.1 gives the analogy between mechanical and electrical quantities.
. So, 1/C is analogous to k. The constant k (=F/x) tells us the (external) force required to produce a unit displacement whereas 1/C (=V/q) tells us the potential difference required to store a unit charge. Table 7.1 gives the analogy between mechanical and electrical quantities.
Note that the above discussion of LC oscillations is not realistic for two reasons:
(i) Every inductor has some resistance. The effect of this resistance is to introduce a damping effect on the charge and current in the circuit and the oscillations finally die away.
(ii) Even if the resistance were zero, the total energy of the system would not remain constant. It is radiated away from the system in the form of electromagnetic waves (discussed in the next chapter). In fact, radio and TV transmitters depend on this radiation.