- 1(current)
Q. No.| 1. | \(4\sqrt2~\text{ms}^{-1},45^\circ\) | 2. | \(4\sqrt2~\text{ms}^{-1},60^\circ\) |
| 3. | \(3\sqrt2~\text{ms}^{-1},30^\circ\) | 4. | \(3\sqrt2~\text{ms}^{-1},45^\circ\) |
The position of a particle is given by,
\(\overrightarrow{\text{r}}=(3.0 \text{t} \hat{\text{i}}-2.0 \text{t}^2 \hat{\text{j}}+4.0 \hat{\text{k}} )~\text{m}\)
where \(t\) is in seconds and the coefficients have the proper units for \(\vec{r}\) to be in meters. What is the magnitude and direction of the velocity of the particle at \(t=2.0\) s?
1. \(7.56~ \text{m} \text{s}^{-1},-70^{\circ}\text{ with} ~\text{y} \text{-axis}. \)
2. \(7.56~ \text{m} \text{s}^{-1}, ~70^{\circ}\text{ with} ~\text{x} \text{-axis}. \)
3. \(8.54 ~\text{m} \text{s}^{-1},~70^{\circ}\text{ with} ~\text{y} \text{-axis}. \)
4. \(8.54 ~\text{m} \text{s}^{-1},-70^{\circ}\text{ with} ~\text{x} \text{-axis}. \)
The following are four different relations about displacement, velocity and acceleration for the motion of a particle in general.
| (a) | \(v_{a v}=1 / 2\left[v\left(t_1\right)+v\left(t_2\right)\right]\) |
| (b) | \(v_{{av}}={r}\left({t}_2\right)-{r}\left({t}_1\right) / {t}_2-{t}_1\) |
| (c) | \(r=1 / 2\left[v\left(t_2\right)-v\left(t_1\right)\right]\left({t}_2-{t}_1\right)\) |
| (d) | \({a}_{{av}}=v\left({t}_2\right)-v\left({t}_1\right) / {t}_2-{t}_1\) |
The incorrect alternative/s is/are:
| 1. | (a), (d) | 2. | (a), (c) |
| 3. | (b), (c) | 4. | (a), (b) |
In a two-dimensional motion, instantaneous speed \(v_0\) is a positive constant. Then which of the following is necessarily true?
| 1. | the average velocity is not zero at any time. |
| 2. | average acceleration must always vanish. |
| 3. | displacements in equal time intervals are equal. |
| 4. | equal path lengths are traversed in equal intervals. |
The position of a particle at time \(t\) is given by, \(x=3t^3\), \(y=2t^2+8t\), and \(z=6t-5\). The initial velocity of the particle is:
| 1. | \(20\) unit | 2. | \(10\) unit |
| 3. | \(5\) unit | 4. | \(13\) unit |
Consider a square carrom board \(ABCD\) of size \({3~ \text{ft}} \times 3~\text{ft}.\) A piece moves 'from' pocket \(A\) (close from a pocket), strikes side \(BC\) and then side \(AD\), and reaches pocket \(C\). If the piece is reflected perfectly from each side, then the ratio of the \(x,y\) components of velocity is given by \(\dfrac{v_x}{v_y}=\)
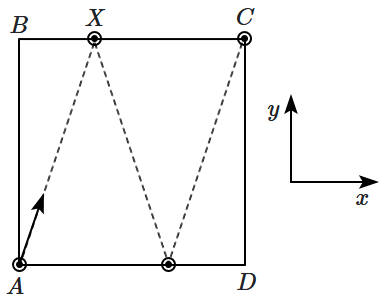
| 1. | \(2\) | 2. | \(\dfrac{1}{2}\) |
| 3. | \(3\) | 4. | \(\dfrac{1}{3}\) |
The coordinates of a moving particle at any time \(t\) are given by \(x=\alpha t^3\) and \(y=\beta t^3.\) The speed of the particle at time \(t\) is given by:
1. \(\sqrt{\alpha^2+\beta^2}~\)
2. \(3t\sqrt{\alpha^2+\beta^2}~\)
3. \(3t^2\sqrt{\alpha^2+\beta^2}~\)
4. \(t^2\sqrt{\alpha^2+\beta^2}~\)
| 1. | \(4~\text{m/s}^2,\) \(2~\text{m/s}\) |
| 2. | \(2~\text{m/s}^2,\) \(4~\text{m/s}\) |
| 3. | \(6~\text{m/s}^2,\) \(2~\text{m/s}\) |
| 4. | \(2~\text{m/s}^2,\) \(6~\text{m/s}\) |
1. \(4~\text{m/s},\) \(53^\circ\) with \(x\)-axis
2. \(4~\text{m/s},\) \(37^\circ\) with \(x\)-axis
3. \(5~\text{m/s},\) \(53^\circ\) with \(y\)-axis
4. \(5~\text{m/s},\) \(53^\circ\) with \(x\)-axis
- 1(current)
Q. No.
© 2024 GoodEd Technologies Pvt. Ltd.