A solid sphere, a hollow sphere, and a disc, all having the same mass and radius, are placed at the top of a smooth incline and released. Least time will be taken in reaching the bottom by 

1.  the solid sphere

2.  the hollow sphere

3.  the disc

4.  all will take the same time  

 

If the kinetic energy of a body increases by 0.1%, the percent increase of its momentum will be 

1.  0.05%

2.  0.1%

3.  1.0%

4.  10%

A solid cylinder and a hollow cylinder, both of the same mass and same external diameter are released from the same height at the same time on an inclined plane. Both roll down without slipping. Which one will reach the bottom first?

1.  Both together only when the angle of inclination of the plane is ${45}^0$

2.  Both together

3.  Hollow cylinder

4.  Solid cylinder

One circular ring and one circular disc both having the same mass and radius. The ratio of their moments of inertia about the axes passing through their centres and perpendicular to planes will be

1. 1:1

2. 2:1

3. 1:2

4. 4:1

A wheel of radius R rolls on the ground with a uniform velocity v. The velocity of topmost point relative to the bottommost point is

1. v

2. 2v

3. v/2

4. zero

The figure shows a uniform solid block of mass M and edge lengths a, b and c. Its M.O.I. about an axis through one edge and perpendicular (as shown) to the large face of the block is 

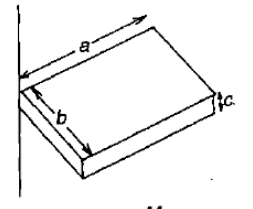
1. $\frac{M}{3}\left(a^2+b^2\right)$

2. $\frac{M}{4}\left(a^2+b^2\right)$

3. $\frac{7M}{12}\left(a^2+b^2\right)$

4. $\frac{M}{12}\left(a^2+b^2\right)$

If the net external forces acting on the system of particles is zero, then which of the following may vary ?

1. Momentum of the system

2. Velocity of centre of mass

3. Position of centre of mass

4. None of the above

A constant torque of 1000 N-m turns a wheel of moment of inertial 200 $\mathrm{kg}-{\mathrm{m}}^2$ about an axis through its centre. Its angular velocity after 3 s is

1. 1 rad/s

2. 5 rad/s

3. 10 rad/s

4. 15 rad/s

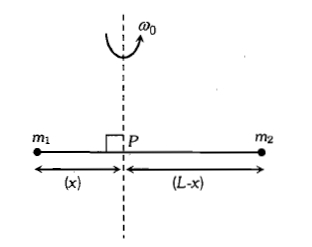
Point masses ${\mathrm{m}}_1$ and ${\mathrm{m}}_2$ are placed at the opposite ends of a rigid of length L and negligible mass. The rod is to be set rotating about an axis perpendicular to it. The position of point P on this rod through which the axis should pass so that the work required to set the rod rotating with angular velocity ${\mathrm{\omega }}_0$ is minimum is given by

$1. \mathrm{x} =\frac{{\mathrm{m}}_1}{{\mathrm{m}}_2}\mathrm{L}$
$2. \mathrm{x} = \frac{{\mathrm{m}}_2}{{\mathrm{m}}_1}\mathrm{L}$
$3. \mathrm{x} = \frac{{\mathrm{m}}_2\mathrm{L}}{{\mathrm{m}}_1+ {\mathrm{m}}_2}$
$4. \mathrm{x} = \frac{{\mathrm{m}}_1\mathrm{L}}{{\mathrm{m}}_1+ {\mathrm{m}}_2}$

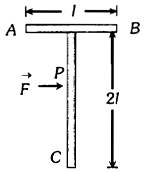
A 'T' shaped object with dimensions shown in the figure, is lying on a smooth floor. A force '$\overrightarrow{\mathrm{F}}$' is applied at the point P parallel to AB, such that the object has only the translational motion without rotation. Find the location of P with respect to C

1.  \(\frac{4}{3} l\)

2.  \(l\)

3.  \(\frac{2}{3} l\)

4.  \(\frac{3}{2} l\)