The following diagram shows a glass sphere of radius 10 cm with a paraxial incident ray. The refractive index of the material of the glass is
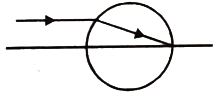
1. 2
2. 1.5
3. 1.75
4. 1.3

A convex lens of focal length 10 cm form real image of an object. The distance between the object and the image may be:
(1) 25 cm
(2) 30 cm
(3) 38 cm
(4) 50 cm
| 1. | \(45\) cm | 2. | \(30\) cm |
| 3. | \(15\) cm | 4. | \(25\) cm |
| 1. | \(X+Y\) | 2. | \(\dfrac{X +Y}{2}\) |
| 3. | \(X-Y\) | 4. | \(\dfrac{X -Y}{2}\) |
Two plane mirrors are placed perpendicular to each other as shown in the figure. An object O is kept at the angular bisector of the two mirrors. Numbers of images of object O is formed, will be:
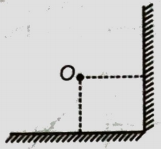
1. 4
2. 3
3. 2
4. 1
Two convex lenses of focal lengths 10 cm and 30 cm are kept at a separation of 20 cm. Then the correct statement is:
(1) The effective focal length is 15 cm
(2) Chromatic aberration is minimized
(3) Combination behaves like a convergent lens
(4) All of these
If one surface of a biconvex lens is silvered, then it behaves as
(1) A concave mirror of focal length
(2) A concave mirror of focal length
(3) A convex mirror of focal length
(4) A concave mirror of focal length
An astronomical telescope has an angular magnification of 20 in its normal adjustment. The focal length of the eyepiece is 4 cm. Distance between objective and eyepiece is:
1. 80 cm
2. 84 cm
3. 76 cm
4. 90 cm
| 1. | a convergent with power \(P=\frac{1}{3 R}\) |
| 2. | a convergent with power \(P=\frac{1}{6 R}\) |
| 3. | a divergent with power \(P=\frac{1}{3 R}\) |
| 4. | a divergent with power \(P=\frac{1}{6 R}\) |
The thickness of a plano-convex glass lens in the middle is 1.5 cm. Viewing from the flat side the thickness of the lens at the centre will appear (refractive index of glass is 1.5) to be
1. 1.5 cm
2. 1.0 cm
3. 2 cm
4. 0.5 cm






