In a uniform magnetic field, a ring is rotating about its axis which is parallel to the magnetic field and the magnetic field is perpendicular to the plane of the ring. The induced electric field in the ring:

1.	Is zero.
2.	Depends on the radius of the ring.
3.	Depends on the nature of the material of the ring.
4.	Depends on the product of the magnetic field and speed.

Which of the following is not based on the application of eddy currents?

1. Speedometer

2. Dead-beat galvanometer

3. Energy meter

4. Tangent galvanometer

A railway track running north-south has two parallel rails l distance apart. If H is the horizontal component of the earth's field, angle of dip is $\theta$, then the induced emf between the rails when a train passes with velocity v, is

1. Hlvtan$\theta$

2. Hlv

3. Hlvcos$\theta$

4. Hlvsin$\theta$

Two coils of self-inductance L₁ and L₂ are placed so close together that they completely link the magnetic flux of each other. If M be their mutual inductance then, the correct relation is 

1. M = $\sqrt{\frac{L_1}{L_2}}$

2. M = L₁$\sqrt{L_2}$

3. M = L₁L₂

4. M = $\sqrt{L_1{L}_2}$

Calculate the self-inductance of a solenoid having \(1000\) turns and length \(1\) m. (The area of cross-section is \(7\) cm² and \(\mu_r=1000).\)

1. \(888\) H

2. \(0.88\) H

3. \(0.088\) H

4. \(88.8\) H

Two discs of radii R and 2R are moving with velocities v and 2v in opposite directions on a conducting platform, as shown in the figure. If magnetic field B is applied in the plane perpendicular to the discs, then the potential difference between two points P and Q which are the topmost points of the discs is 

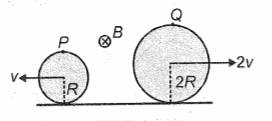
1. 8BRv

2. 4BRv

3. 10BRv

4. 2BRv

What is the effective self-inductance of two solenoids in series-wound on a core as shown in the figure, if L is the self-inductance of each solenoid?

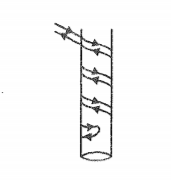
1. L

2. 2L

3. L/2

4. Zero

A uniform conductor having length l is moving in uniform magnetic field B with speed v. If B, l and v are mutually perpendicular and R is the resistance of the conductor, then the power delivered by the external source is

1. B²l²v²/2R

2. 2B²l²v²/R

3. B²l²v²/R

4. Zero

A circular ring of radius \(r\) is at the centre of a square having side  \(a\left ( a\gg r \right ).\) The mutual inductance of the system is:
1. \( \dfrac{\mu_0 r^2}{a}\)
2. \( \dfrac{\sqrt{2} \mu_0 r^2}{\pi} \)
3. \(\dfrac{2 \sqrt{2} \mu_0 r^2}{a}\)
4. \( \dfrac{2 \sqrt{2} \mu_0 r^2}{\pi a}\)

If a semicircular conducting loop \(ACB\) is rotating in the plane of paper with constant angular velocity \(\omega\) about \(O\), then which of the following graphs shows the variation of induced current \((I)\) with time \((t)\)? The magnetic field is perpendicular to the plane of the loop as shown in the figure.
               

1.		2.
3.		4.