Let $\theta$ denote the angular displacement of a simple pendulum oscillating in a vertical plane. If the mass of bob is m, the tension in the string is mgcos$\theta$-

1.  always 

2.  never

3.  at extreme

4.  at mean position

The graph for stopping time (t) versus mass (m) is shown in the figure. Then which one of the pairs is given?

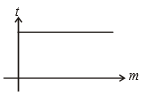
1.  Momentum, force

2.  K.E., force

3.  Velocity, force

4.  None of these

A metal ring of mass m and radius R is placed on smooth horizontal table and is set rotating about its own axis in such a way that each part of the ring moves with a speed v. Tension in the ring is:

1. $\frac{m{v}^2}{R}$

2. $\frac{m{v}^2}{2R}$

3. $\frac{m{v}^2}{2\mathrm{\pi }R}$

4. None of these

Tension on the string at point P is T. The graph for tension (T) versus x is shown in the figure. Then the string is:

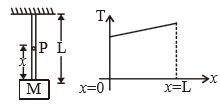
1.  massless

2.  massfull

3.  tension on every point on the string is same when the string is having finite mass 4

4.  None of these

While walking on ice one should take small steps to avoid slipping. This is because smaller steps ensure

1. Larger friction

2. Smaller friction

3. Larger normal force

4. Smaller normal force

A block is kept on a rough inclined plane with angle of inclination $\theta$. The graph of net reaction (R) versus $\theta$ is:

1.		2.
3.		4.	None of these

1. 2. 

3. 4. None of these

A block is placed on a rough horizontal plane. A time dependent horizontal force F=kt acts on the block. The acceleration time graph of the block is :

1.		2.
3.		4.

1. 2. 

3. 4. 

A motorcycle is going on an overbridge of radius R. The driver maintains a constant speed. As the motorcycle is ascending on the overbridge, the normal force on it:

1.  Increases

2.  decreases

3.  remains the same

4.  fluctuates erratically

A rod of length L and mass m is acted on by two unequal forces $F_1$ and $F_2\left(<F_1\right)$ as shown in the following figure

The tension in the rod at a distance y from the end A is given by :

1. $F_1\left(1-\frac{y}{L}\right)+F_2\left(\frac{y}{L}\right)$

2. $F_2\left(1-\frac{y}{L}\right)+F_1\left(\frac{y}{L}\right)$

3. $\left(F_1-F_2\right)\frac{y}{L}$

4. None of the above

Two blocks A and B of masses m & 2m respectively are held at rest such that the spring is in natural length. Find the accelerations of both the blocks just after release.

1. \(g \downarrow ,   g \downarrow\)

2. \(\frac{g}{3} \downarrow ,   \frac{g}{3} \uparrow\)

3. (0, 0)

4. \(g \downarrow ,   0\)