The radii of curvature of the faces of a double convex lens are \(10\) cm and \(15\) cm. Its focal length is \(12\) cm. What is the refractive index of glass?
1. \(1.3\)
2. \(1.2\)
3. \(1.5\)
4. \(1.4\)
1. \(1.3\)
2. \(1.2\)
3. \(1.5\)
4. \(1.4\)
A convex lens has a \(20~\text{cm}\) focal length in air. What is the focal length in water?
(the refractive index of air-water \(=1.33,\) refractive index for air-glass \(=1.5\))
1. \(+72.72~\text{cm}\)
2. \(+78.2~\text{cm}\)
3. \(-72.72~\text{cm}\)
4. \(+70.2~\text{cm}\)
Find the position of the image formed by the lens combination in the given figure.

The final image is:
| 1. | formed \( 30 ~\text{cm} \) to the right of the third lens. |
| 2. | formed \(20 ~\text{cm} \) to the left of the third lens. |
| 3. | formed \( 30 ~\text{cm} \) to the left of the third lens. |
| 4. | formed \(20 ~\text{cm} \) to the right of the third lens. |
What focal length should the reading spectacles have for a person for whom the least distance of distinct vision is \(50~\text{cm}\)?
1. \(-50~\text{cm}\)
2. \(+50~\text{cm}\)
3. \(-60~\text{cm}\)
4. \(+60~\text{cm}\)
The far point of a myopic person is \(80~\text{cm}\) in front of the eye. What is the power of the lens required to enable him to see very distant objects clearly?
1. \(-1.25~\text{D}\)
2. \(+1.25~\text{D}\)
3. \(-2.12~\text{D}\)
4. \(+2.12~\text{D}\)
The near point of a hypermetropic person is \(75~\text{cm}\) from the eye. What is the power of the lens required to enable the person to read clearly a book held at \(25~\text{cm}\) from the eye?
1. \(+2.67~\text{D}\)
2. \(-1.25~\text{D}\)
3. \(-2.67~\text{D}\)
4. \(+1.25~\text{D}\)
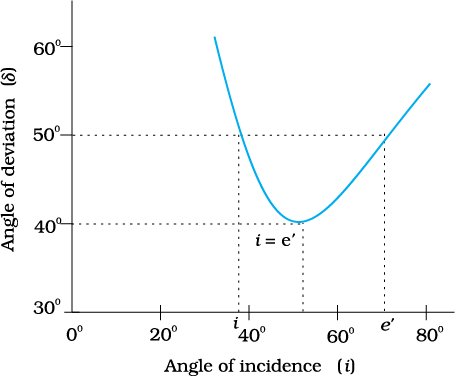
In the diagram given below, plot of angle of deviation () versus angle of incidence (i) for a triangular prism is given. Then the angle of minimum deviation is-
1.
2.
3.
4.
Find the value of the angle of emergence from the prism given below for the incidence ray shown. The refractive index of the glass is \(\sqrt{3}\).
1. \(45^{\circ}\)
2. \(90^{\circ}\)
3. \(60^{\circ}\)
4. \(30^{\circ}\)
A lens of large focal length and large aperture is best suited as an objective of an astronomical telescope since:
| 1. | a large aperture contributes to the quality and visibility of the images. |
| 2. | a large area of the objective ensures better light-gathering power. |
| 3. | a large aperture provides a better resolution. |
| 4. | all of the above. |
A point object is placed at a distance of \(60~\text{cm}\) from a convex lens of focal length \(30~\text{cm}\). If a plane mirror were put perpendicular to the principal axis of the lens and at a distance of \(40~\text{cm}\) from it, the final image would be formed at a distance of:

| 1. | \(30~\text{cm}\) from the plane mirror, it would be a virtual image. |
| 2. | \(20~\text{cm}\) from the plane mirror, it would be a virtual image. |
| 3. | \(20~\text{cm}\) from the lens, it would be a real image. |
| 4. | \(30~\text{cm}\) from the lens, it would be a real image. |







