The de-Broglie wavelength of a body of mass 1 kg moving with a velocity of 2000 m/s is

1.  $3.32 \mathrm{x} {10}^{-27} \stackrel{\mathrm{o}}{\mathrm{A}}$

2.  $1.5 \mathrm{x} {10}^7 \stackrel{\mathrm{o}}{\mathrm{A}}$

3.  $0.55 \mathrm{x} {10}^{-22} \stackrel{\mathrm{o}}{\mathrm{A}}$

4.  None of these

Light of wavelength 4000 Å falls on a photosensitive metal and a negative 2V potential stops the emitted electrons.

The work function of the material (in eV) is approximately 

$\left(\mathrm{h}=6.6 \mathrm{x} {10}^{-34} \mathrm{Js}, \mathrm{e}=1.6 \mathrm{x} {10}^{-19} \mathrm{C}, \mathrm{c}=3 \mathrm{x} {10}^8 {\mathrm{ms}}^{-1}\right)$
1.  1.1

2.  2.0

3.  2.2

4.  3.1

The work functions for metal A, metal B, and metal C are ${\varphi }_A,{\varphi }_B and {\varphi }_C$ respectively. Then-

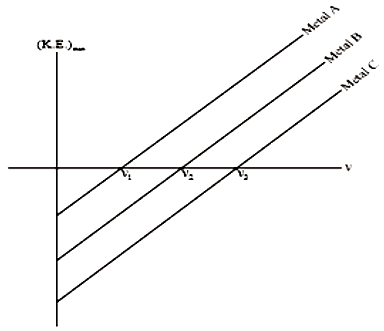
1.   ${\mathrm{\varphi }}_{\mathrm{A}} < {\mathrm{\varphi }}_{\mathbf{B}} < {\mathrm{\varphi }}_{\mathrm{C}}$

2.  ${\mathrm{\varphi }}_{\mathrm{A}} = {\mathrm{\varphi }}_{\mathbf{B}} < {\mathrm{\varphi }}_{\mathrm{C}}$

3.  ${\mathrm{\varphi }}_{\mathrm{A}} > {\mathrm{\varphi }}_{\mathbf{B}} > {\mathrm{\varphi }}_{\mathrm{C}}$

4.  None of these

An electron at rest is acceleration by a potential difference of 600V. The de-Broglie wavelength associated with the electron is:

1.  $1\stackrel{o}{A}$

2.  $2\stackrel{o}{A}$

3.  $3\stackrel{o}{A}$

4.  $0.5 \stackrel{o}{A}$

For non-relativistic speeds, the wavelength associated with an electron and its kinetic energy E are related as:

1.  $\mathrm{\lambda } \propto \mathrm{E}$

2.  $\mathrm{\lambda } \propto {\mathrm{E}}^{-1}$

3.  $\mathrm{\lambda } \propto {\mathrm{E}}^{1/2}$

4.  $\mathrm{\lambda } \propto {\mathrm{E}}^{-1/2}$

If the momentum of a photon is p, then its energy is :

1.  pc

2.  $\frac{1}{2}\mathrm{pc}$

3.  $\frac{{\mathrm{p}}^2}{2\mathrm{c}}$

4.  $\frac{1}{4}\mathrm{pc}$

When the light of wavelength $\mathrm{\lambda }$ is used in a photoelectric experiment, the maximum kinetic energy of emitted electrons is found to be K. What will the maximum kinetic energy of the photoelectrons when the light of wavelength $\frac{\mathrm{\lambda }}{2}$ is used instead of $\mathrm{\lambda }$:

1. Zero

2. Non zero but less than 2 K

3. 2K

4. More than 2K

Electrons used in an electron microscope are accelerated by a voltage of 25 kV. If the accelerating voltage is reduced to 6.25 kV, the resolving power of the microscope would:

1. Increase to 4 time

2. Increases to 2 times

3. Decrease to half

4. Decrease to 1/4th

The ratio of wavelengths of the last line of Balmer series and the last line of Lyman series is: 

(1) 2

(2) 1

(3) 4

(4) 0.05