A sphere of 4 cm radius is suspended within a hollow sphere of 6 cm radius. The inner sphere is charged to potential 3 e.s.u. and the outer sphere is earthed. The charge on the inner sphere is 

(1) 54 e.s.u.

(2) $\frac{1}{4}$ e.s.u.

(3) 30 e.s.u.

(4) 36 e.s.u.

When one electron is taken towards the other electron, then the electric potential energy of the system -

(1) Decreases

(2) Increases

(3) Remains unchanged

(4) Becomes zero

Four charges $+Q,-Q,+Q,-Q$ are placed at the corners of a square taken in order. At the centre of the square 

(1) $E=0,V=0$

(2) $E=0,V\ne 0$

(3) $E\ne 0,V=0$

(4) $E=0,V\ne 0$

Point charge q₁ = 2 μC and q₂ = –1 μC are kept at points x = 0 and x = 6 respectively. Electrical potential will be zero at points 

(1) x = 2 and x = 9

(2) x = 1 and x = 5

(3) x = 4 and x = 12

(4) x = –2 and x = 2

Equipotential surfaces associated with an electric field which is increasing in magnitude along the x-direction are 

(1) Planes parallel to yz-plane

(2) Planes parallel to xy-plane

(3) Planes parallel to xz-plane

(4) Coaxial cylinders of increasing radii around the x-axis

A bullet of mass 2 gm is having a charge of 2 μC. Through what potential difference must it be accelerated, starting from rest, to acquire a speed of 10 m/s ?

(1) 5 kV

(2) 50 kV

(3) 5 V

(4) 50 V

In a certain charge distribution, all points having zero potential can be joined by a circle \(S.\) The points inside \(S\) have positive potential, and points outside \(S\) have a negative potential. A positive charge, which is free to move, is placed inside \(S.\) What is the correct statement about \(S\):

A square of side ‘a’ has charge Q at its centre and charge ‘q’ at one of the corners. The work required to be done in moving the charge ‘q’ from the corner to the diagonally opposite corner is -

(1) Zero

(2) $\frac{Qq}{4\pi {\in }_{0}a}$

(3) $\frac{Qq\sqrt{2}}{4\pi {\in }_{0}a}$

(4) $\frac{Qq}{2\pi {\in }_{0}a}$

As per this diagram a point charge +q is placed at the origin O. Work done in taking another point charge –Q from the point A [co-ordinates (0, a)] to another point B [co-ordinates (a, 0)] along the straight path AB is

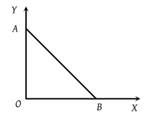
(1) Zero

(2) $\left(\frac{{\displaystyle -qQ}}{{\displaystyle 4\pi {\epsilon }_0}}\frac{{\displaystyle 1}}{{\displaystyle a^2}}\right)\sqrt{2}a$

(3) $\left(\frac{{\displaystyle qQ}}{{\displaystyle 4\pi {\epsilon }_0}}\frac{{\displaystyle 1}}{{\displaystyle a^2}}\right)\frac{a}{\sqrt{2}}$

(4) $\left(\frac{{\displaystyle qQ}}{{\displaystyle 4\pi {\epsilon }_0}}\frac{{\displaystyle 1}}{{\displaystyle a^2}}\right)\sqrt{2}a$

Two charges \(q_1\) and \(q_2\) are placed \(30~\text{cm}\) apart, as shown in the figure. A third charge \(q_3\) is moved along the arc of a circle of radius \(40~\text{cm}\) from \(C\) to \(D.\) The change in the potential energy of the system is \(\dfrac{q_{3}}{4 \pi \varepsilon_{0}} k,\) where \(k\) is: