Point charge q₁ = 2 μC and q₂ = –1 μC are kept at points x = 0 and x = 6 respectively. Electrical potential will be zero at points 

1. x = 2 and x = 9

2. x = 1 and x = 5

3.  x = 4 and x = 12

4. x = –2 and x = 2

Equipotential surfaces associated with an electric field which is increasing in magnitude along the x-direction are 

1. Planes parallel to yz-plane

2. Planes parallel to xy-plane

3. Planes parallel to xz-plane

4. Coaxial cylinders of increasing radii around the x-axis

A bullet of mass 2 gm is having a charge of 2 μC. Through what potential difference must it be accelerated, starting from rest, to acquire a speed of 10 m/s ?

1. 5 kV

2. 50 kV

3.  5 V

4. 50 V

Figure shows three points A, B and C in a region of uniform electric field $\overrightarrow{E}$. The line AB is perpendicular and BC is parallel to the field lines. Then which of the following holds good. Where $V_A,V_B$ and V_C represent the electric potential at points A, B and C respectively

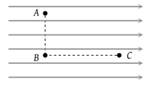
1. $V_A=V_B=V_C$

2. $V_A=V_B>V_C$

3. $V_A=V_B<V_C$

4. $V_A>V_B=V_C$

In a certain charge distribution, all points having zero potential can be joined by a circle \(S.\) The points inside \(S\) have positive potential, and points outside \(S\) have a negative potential. A positive charge, which is free to move, is placed inside \(S.\) What is the correct statement about \(S\):

A square of side ‘a’ has charge Q at its centre and charge ‘q’ at one of the corners. The work required to be done in moving the charge ‘q’ from the corner to the diagonally opposite corner is -

1. Zero

2. $\frac{Qq}{4\pi {\in }_{0}a}$

3. $\frac{Qq\sqrt{2}}{4\pi {\in }_{0}a}$

4. $\frac{Qq}{2\pi {\in }_{0}a}$

As per this diagram a point charge +q is placed at the origin O. Work done in taking another point charge –Q from the point A [co-ordinates (0, a)] to another point B [co-ordinates (a, 0)] along the straight path AB is

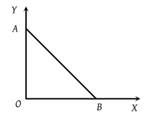
1. Zero

2. $\left(\frac{{\displaystyle -qQ}}{{\displaystyle 4\pi {\epsilon }_0}}\frac{{\displaystyle 1}}{{\displaystyle a^2}}\right)\sqrt{2}a$

3. $\left(\frac{{\displaystyle qQ}}{{\displaystyle 4\pi {\epsilon }_0}}\frac{{\displaystyle 1}}{{\displaystyle a^2}}\right)\frac{a}{\sqrt{2}}$

4. $\left(\frac{{\displaystyle qQ}}{{\displaystyle 4\pi {\epsilon }_0}}\frac{{\displaystyle 1}}{{\displaystyle a^2}}\right)\sqrt{2}a$

Two charges \(q_1\) and \(q_2\) are placed \(30~\text{cm}\) apart, as shown in the figure. A third charge \(q_3\) is moved along the arc of a circle of radius \(40~\text{cm}\) from \(C\) to \(D.\) The change in the potential energy of the system is \(\dfrac{q_{3}}{4 \pi \varepsilon_{0}} k,\) where \(k\) is:

Two thin wire rings each having a radius R are placed at a distance d apart with their axes coinciding. The charges on the two rings are +q and –q. The potential difference between the centres of the two rings is -

1. Zero

2. $\frac{Q}{4\pi {\epsilon }_0}\left[\frac{{\displaystyle 1}}{{\displaystyle R}}-\frac{{\displaystyle 1}}{{\displaystyle \sqrt{R^2+d^2}}}\right]$

3. $QR/4\pi {\epsilon }_0{d}^2$

4. $\frac{Q}{2\pi {\epsilon }_0}\left[\frac{{\displaystyle 1}}{{\displaystyle R}}-\frac{{\displaystyle 1}}{{\displaystyle \sqrt{R^2+d^2}}}\right]$

A hollow metallic sphere of radius R is given a charge Q. Then the potential at the centre is -

1. Zero

2. $\frac{1}{4\pi {\epsilon }_0}.\frac{Q}{R}$

3. $\frac{1}{4\pi {\epsilon }_0}.\frac{2Q}{R}$

4. $\frac{1}{4\pi {\epsilon }_0}.\frac{Q}{2R}$