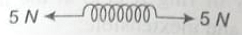A pendulum of mass m hangs from a support fixed to a trolley. The direction of the string when the trolley rolls up a plane of inclination with acceleration is

1.
2.
3.
4.

The pulleys and string shown in the figure are smooth and of negligible mass. For the system to remain in equilibrium, the angle should be:
1. 0°
2. 30
3. 45
4. 60
System shown in figure is in equilibrium and at rest. The spring and string are massless, now the stringis cut. The acceleration of mass 2m and m just after string is cut will be
1. g/2 upwards, g downwards
2. g upwards, g/2 downwards
3. g upwards, 2g downwards
4. 2g upwards, g downwards
In the system shown . System is held at rest by thread BC. Just after lower thread is Burnt.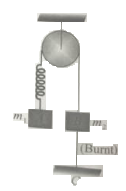
1. Acceleration of is upward
2. Magnitude of acceleration of both blocks will be
3. Acceleration of will be non-zero
4. Magnitude of acceleration of two blocks will be non-zero and unequal.
Two blocks of 4 kg and 6 kg are attached by springs, they are hanging in vertical position, lower spring breaks due to excessive force. Acceleration of 4 kg block just after breaking
1. 15
2. 25
3. 10
4. Zero
A block can slide on a smooth inclined plane of inclination kept on the floor of a lift. When the lift is descending with retardation a, the acceleration of the block relative to the incline is:
1. (g + a) sin
2. (g – a)
3. g sin
4. (g – a) sin
A mass m1, placed on top of a trolley of mass m3, is connected to another mass m2 by means of string passing over a smooth pulley as shown in figure. The friction between surfaces is negligible. For m1 ans m2 not to move with respect to trolley, the horizontal force F to be applied on trolley is
1. F=m3g
2. F=(m1 + m2)g
3.
4. F= m1g
In the shown system, each of the blocks is at rest. The value of is:

1.
2.
3.
4.
A particle moving with velocity is acted by three forces shown by the vector triangle The velocity of the particle will:

| 1. | change according to the smallest force |
| 2. | increase |
| 3. | decrease |
| 4. | remain constant |