For the circuit shown in figure below, the ammeter $A_2$ reads 1.6 A and ammeter $A_3$ read 0.4 A If ${\omega }_0$ is angular frequency and $f$ is frequency of ac, then

$1. {\omega }_0=\frac{4}{\sqrt{LC}}$
$2. f=\frac{2\pi }{\sqrt{LC}}$
$3. The ammeter A_1 reads 1.2 A$
$4. The ammeter A_1 reads 2 A$

Given that the current \(i_1=3A \sin \omega t\) and the current \(i_2=4A \cos \omega t,\) what will be the expression for the current \(i_3\)?

                  
1. \(5 A \sin \left(\omega t+53^{\circ}\right) \)
2. \(5 A \sin \left(\omega t+37^{\circ}\right) \)
3. \(5 A \sin \left(\omega t+45^{\circ}\right) \)
4. \( 5 A \sin \left(\omega t+30^{\circ}\right)\)

In a box \(Z\) of unknown elements (\(L\) or \(R\) or any other combination), an ac voltage \(E = E_0 \sin(\omega t + \phi)\) $$is applied and the current in the circuit is found to be \(I = I_0 \sin\left(\omega t + \phi +\frac{\pi}{4}\right)\). The unknown elements in the box could be:
             

The phase difference between emf and current through the choke coil maybe

1. 0$°$

2. 85$°$

3. 45$°$

4. 30$°$

What is the phase difference between potential difference across the inductor and potential difference across the capacitor in a series LCR circuit?

1. Zero

$2. \frac{\pi }{4}$
$3. \frac{\pi }{4}$
$4. \pi$

What is the reading of the A.C voltmeter in the network as shown in the figure?

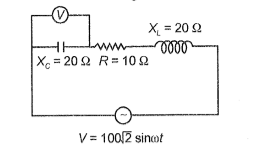
1. zero

2. 100 V

3. 200 V

4. 400 v

When an alternating voltage is given as; \(E = (6 \sin\omega t - 2 \cos \omega t)~\text V,\) what is its RMS value?
1. \(4 \sqrt 2 ~\text V\) 
2. \(2 \sqrt 5 ~\text V\) 
3. \(2 \sqrt 3 ~\text V\) 
4. \(4 ~\text V\) 

An LC circuit contains an inductor (L=25 mH) and a capacitor (C=25 mF) with an initial charge of Q₀. At what time will the circuit have an equal amount of electrical and magnetic energy?

$1.$ $\frac{\mathrm{\pi }}{\mathrm{160 }}s$

$2.$ $\frac{3\mathrm{\pi }}{\mathrm{160 }}s$

$3.$ $\frac{5\mathrm{\pi }}{\mathrm{160 }}s$

4. All of these

In which of the following circuits can the power factor be zero?

1. LC circuit

2. LCR circuit

3. Purely resistive circuit

4. Both (1) & (2)